


The Slepkov Biophotonics Lab group is in the business of conducting original curiosity-based and applied research. The key motivator for most of the research is “curiosity”. Subject area, outcomes, motivation, and respectability are only of secondary concern in choosing a project. Thus, we are conducting research on widespread and disparate topics, which can be grouped into three main categories: Nonlinear Optical Microscopy, Physics Education Research, and Mad Science.
The ability to visualize unique structures and dynamic processes on the micron scale has excited scientists for centuries. When asked to describe the most useful developments in microscopy this century, many would focus on improved resolution—the ability to see smaller and smaller objects. However, it could be argued that the ability to distinguish between objects/regions is equally important to being able to characterize samples of interest. This ability to distinguish, to identify, primarily concerns contrast, not resolution. Thus, creating contrast between structures/compounds of interest and their surroundings is incredibly important. Most major breakthroughs in microscopy in the last quarter century involve discovering or utilizing unique contrast mechanism. Nonlinear optical (NLO) phenomena—those that depend on the intensity of light, rather than just on the wavelength—are now providing an ever-increasing range of unique contrast mechanisms that are particularly suited for studying complex biomaterials.
In terms of NLO microscopy we are primarily interested in label-free stimulated vibrational mode imaging of key structures/molecules, where we continue to develop broadband laser pulse based approaches for implementing coherent Raman scattering techniques such as CARS and SRS. Our primary research interests lie in exploring the utility of such techniques for the advanced materials characterization beyond biomedicine. In particular, we have recently published applications of multimodal CARS microscopy in disciplines such as natural biopolymers (cellulose, starch, chitin), geology (oil/gas deposits in rocks), and aquaculture bio-pharma (carotenoids in algae).
We are establishing world-class experimental capabilities in our laboratory at Trent University (see picture gallery), and are continuing to collaborate with other world-class laboratories such as the National Research Council of Canada (NRC) in Ottawa.

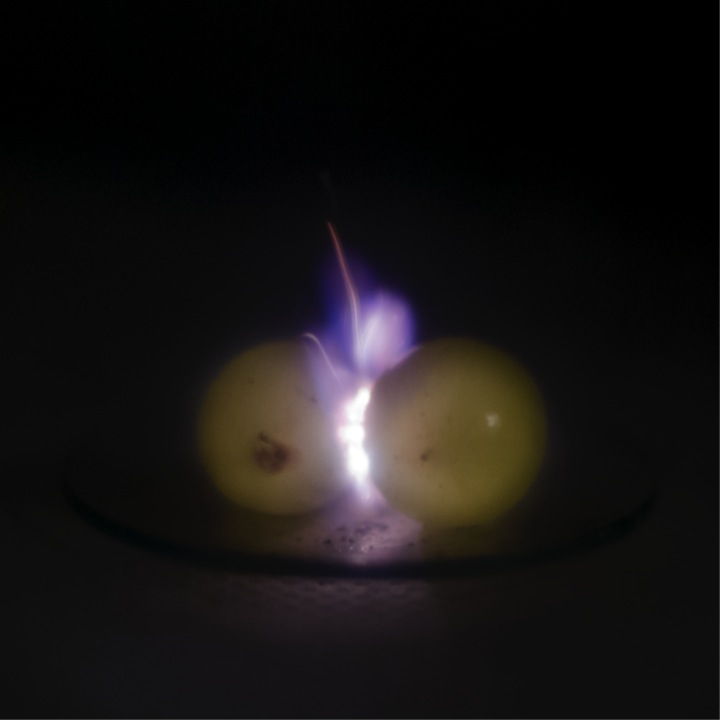
For over fifteen years now, creating a plasma (ball-lightning) from cut grapes in a household microwave oven has been an entertaining, if perplexing, parlour trick. Many explanations abound for why/how the plasma forms in such systems, but there are a few nagging questions and observations that challenge the traditional interpretation. In addition, there are those (not us!) who fear that cellphone and wireless radiation are harmful to biotissue, causing mutations and tumours. While we usually point out that the relevant microwave radiation is non-ionizing, and thus poses no such danger, how can we reconcile this notion with the observation that high-intensity microwave radiation can easily ionize grapes (biotissue)? Our group has been studying this problem and have some very interesting data, including a new explanation for the phenomena. Stay tuned….
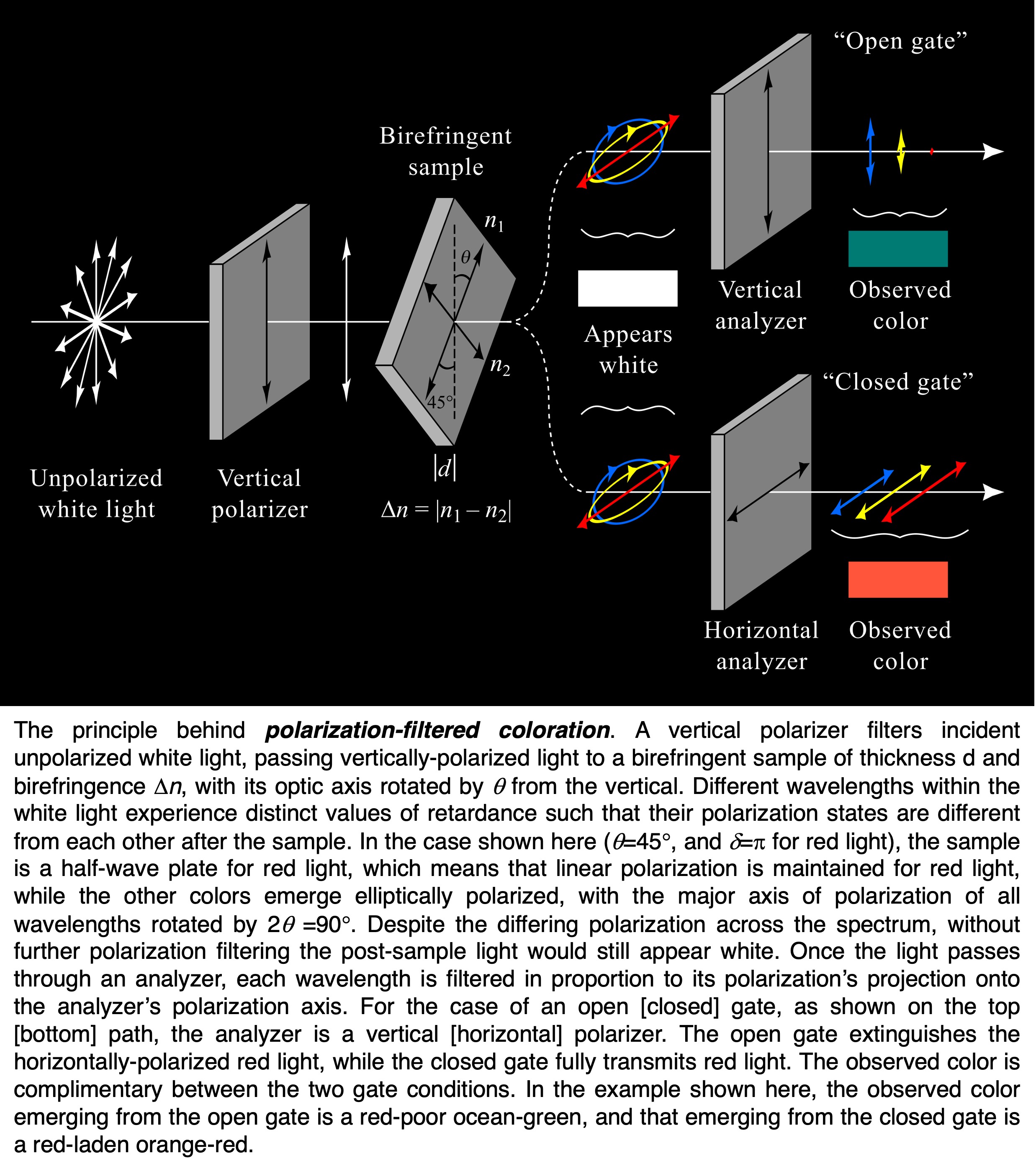
What started as a Twitter-inspired at-home pandemic project has (predictably) led to a rabbit-hole of art, laboratory development, quantitative spectroscopy, and science communication. The variety of colours that can be observed when a birefringent sample is placed between crossed polarizers has been appreciated since the early 1800's and has found useful applications in gemology and geology, glass manufacturing, and modern LCD displays. The phenomenon is commonly referred to as "Interference Colours" but is the reference to interference informative or misleading? Artists call it "polage", but we prefer Polarization Filtered Colouration, or PFC. Read the paper to see why, and to see more nuanced physics associated with order-of-operations, optical path length, and birefringence arithmetic.
We've explored PFC with a bunch of birefringent household films such as scotch tape, kitchen cling wrap, gift basket wrap, and true cellophane. Obviously, any opportunities for the use of these samples for colouration depends on the material birefringence and thickness. Surprisingly, there are almost no reports of quantitative measurements of birefringence in transparent polymers. Our work addresses this by showing a simple method of measuring the birefringence of thin film across the visible spectrum. Incandescent floodlight as a lightsource...no lasers!
Painting in Polarization has already received considerable media attention. See what others have to say about the work:
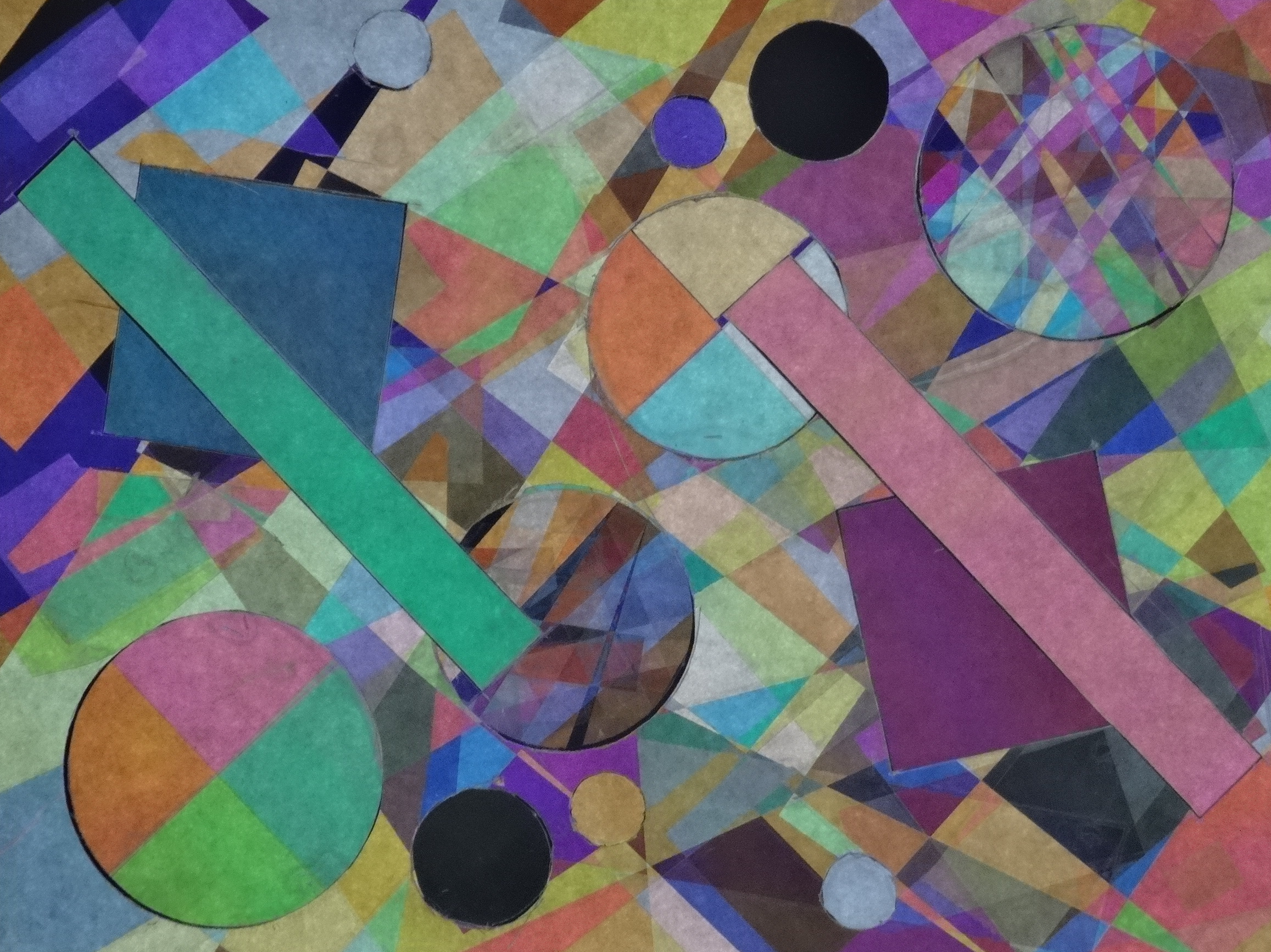
The discipline of physics lies at an interesting academic nexus of mathematics, engineering, and philosophy. As such, the teaching of physics requires student engagement with an enormous set of varied skills; both quantitative and conceptual. Techniques for “teaching” physics have developed across the decades, and in many ways research in physics education has pioneered revolutions in pedagogical approaches across disciplines. Course content delivery is often the primary concern of traditional PER. Assessment (tests, quizzes, exams) has traditionally been of secondary concern in our discipline. It is often thought of as a dirty necessity that can be more art than science. In the last few decades, fundamental changes in the way we administer assessments in physics courses have been driven by economic pragmatism—often at odds with pedagogical motivations.
Assessment is our main PER interest. We are studying why and how it is that we assess students in physics, and how this compares to other disciplines. We are then also developing new assessment structures that target pedagogical deficiencies (or needs) in physics education, but which then can be applied to other STEM and non-STEM disciplines. In particular, we are developing unique multiple-choice question structures that utilize answer-until-correct response formats to assess the integration of skills and concepts that span the cognitive domain. Such groups of questions, denoted Integrated Testlets (ITs), are being developed as assessment tools that address (or even enhance) both our pedagogical and economic needs.
In our lab we both developing integrated testlets, and exploring/creating statistical and psychometric tools for assessing the ITs themselves. As in all things we do, we foremost take a scientific approach to answering the questions of what the tools do, and how it is that we know what they do.
Do you ever ask a simple question only to find that no one has an adequate answer? Do you ever ask a stupid question only to find that the answer is more nuanced or interesting than you first thought? We ask these kinds of questions all the time. The key is identifying when we should explore further the answers to such questions. Another key is following our curiosity first and worrying about how to frame our findings later. Our ethos is that as long as we are continually challenging our current assumptions, we should continue to dig deeper until we either run out curiosity or until the projected value of the answer is outmatched by the projected cost of finding out. Of course, once we have satisfied our own curiosity, we endeavour to publish our findings in high-visibility peer-reviewed journals. Over the past few years we have undertaken several one-off projects that best fit the above description. These projects are tangentially linked to biophotonics or to education research, but do not squarely fit in either. Below are a couple of examples:
Benford’s law describes the logarithmic (i.e NOT UNIFORM) distribution of leading digits prevalent in a wide array of data sets and number sequences. Because we are interested in developing better multiple-choice question formats, it is important for us to assure that multiple-choice testing as an assessment tool is itself robust. An alcohol-fuelled discussion once raised the spectre that the printed answers in the back of physics textbooks might conform to Benford’s Law, and that if this is indeed true, might the answers to numeric multiple-choice questions also follow this pattern. If so, might Benford’s Law be used to gain advantage over random guessing in numerical multiple choice? For the answer, read the research article, or the MIT Technology Review story (or APS blog) to find out more.